CHAPTER 4 PRICE INDEX THEORY
OVERVIEW
4.1 This chapter describes the price index theory underpinning the HPI. A more comprehensive exploration of price index theory can be found in corresponding chapters of Information Paper: Consumer Price Index: Concepts, Sources and Methods, 2009 (cat. no. 6461.0) and Producer and International Trade Price Indexes: Concepts, Sources and Methods, 2006 (cat. no. 6429.0).
4.2 As the name indicates, the HPI is presented as a price index. Price indexes provide a convenient and consistent way of presenting price movement information that overcomes problems associated with averaging across diverse items. An index number on its own has little meaning. The value of a price index stems from the fact that index numbers for any two periods can be used to directly calculate price change between those periods. For example, the HPI Sydney index number of 97.2 in December quarter 2008 informs the user that Sydney house prices have fallen by 2.8% from the base year 2003-04 (when the index was set to 100.0).
CONCEPT OF A PRICE INDEX
Comparing prices
4.3 There are many situations where there is a need to compare two (or more) sets of observations on prices. For example, households may want to compare prices of goods and services today with an earlier time period, and therefore could refer to the CPI. With regard specifically to house prices, a home buyer may want to be able to compare movements in a city over time, or an economist may want to compare house price movements between countries over time to analyse a country's economic performance.
4.4 The most common comparison is between sets of prices at two times (temporal indexes). The times can be adjacent or many periods apart. Another application is to compare prices between regions or countries for the same time (spatial indexes).
4.5 In some situations, the price comparisons might only involve a single commodity. Here it is simply a matter of directly comparing the two price observations. For example a family selling their house might want to assess how the price recommended by their real estate agent compares with the price when they purchased it.
4.6 In other circumstances, the required comparison is of prices across a range of commodities. Although comparisons can readily be made for individual or identical items, a method is required for combining prices across a diverse range of items allowing for differences in the items. For the CPI or Producer Price Index (PPI), items may come in different units or quantities of measurement. In the context of the HPI, the composition of the set of houses sold every quarter varies in terms of location and physical characteristics.
4.7 For HPIs, there are a variety of methodological approaches for addressing this issue of compositional change in the derivation of representative prices or price movements (see Chapter 5). Further, for the HPI as for the CPI and PPI, price indexes play an important role in combining these prices or movements into aggregate measures.
The basic concept
4.8 A price index is a measure of changes in a set of prices over time. Price indexes allow the comparison of two sets of prices for a common item or group of items. In order to compare sets of prices, it is necessary to designate one set the 'reference' set and the other the 'comparison' set. The reference price set is used as the 'base' period for constructing the index and is generally given an index value of 100.0.
4.9 For example, suppose for a single item the average of prices in a set (set 1) was $300,000 and for set 2 was $600,000. Then designating set 1 as the reference set gives an index of 200.0 (600,000 / 300,000 x 100) for the comparison set 2. Designating set 2 as the reference set gives an index of 50.0 (300,000 / 600,000 x 100) for set 1.
4.10 The method for aggregating and comparing price change across a range of commodities usually involves values.
4.11 Values of sales of products are determined by both the prices of the goods or services and the quantities of the goods or services being sold. Therefore, by fixing the quantities of the different items being measured, the change in value in a certain period will be attributable to the change in prices of the items. In CPI or PPI terms, the fixed quantities are called a 'basket'. In the HPI, corresponding references are to the 'housing stock'.
4.12 Beginning with the fixed quantities, price indexes can be constructed for different points in time. Typically, the method is to nominate one set of prices as the reference prices and to revalue the quantities of items purchased in the reference period by prices in the second period. The ratio of the revalued housing stock, in the case of the HPI, to the value of the housing stock in the reference period provides a measure of the price change between the two periods.
REFINING THE CONCEPT
4. 13 The value of an individual item is the product of price and quantity:
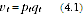
where v is value, p is price, and q is quantity, and the subscript t refers to the periods at which the observations are made.
4.14 Changes in the value of the same item at different points in time can reflect changes in the actual price, changes in the quantity involved, or a combination of both price and quantity changes. Decomposition of a change in a value can be illustrated using equation 4.1, as in the following example.
4.15 Suppose the price of representative houses in a particular suburb are $200,000 at a particular time. Suppose further that the price rises to $250,000 at a later time. The movement in the price of houses from the first to the later period is obtained from the ratio of the price in the second period to the price in the first period, that is $250,000/$200,000 = 1.25 or an increase of 25% in the price. If exactly the same quantity of houses were sold in the two periods, the value of the sales would rise by 25%. However, if the number sold in the first period was 10 houses, and the number sold in the second period was 12 houses, the quantity would also have risen, by 12/10 = 1.20 or 20%. In these circumstances, the total value of sales of houses increases from $2,000,000 in the first period (10 houses at $200,000 per house), to $3,000,000 in the second period (12 houses at $250,000 per house), an overall increase in value of $1,000,000, or 50%. The overall increase in value is the product of the ratios of the change in price and the change in quantity (1.25 x 1.20 = 1.50).
4.16 For an individual item, the ratio between the price in the current period and the price in the reference period is called a price relative. A price relative shows the change in price for one item only.
4.17 In terms of the formula in equation 4.1:
v1= p1 ($200,000) ×q1 (10 houses) = $2,000,000 and
v2= p2 ($250,000) ×q2 (12 houses) = $3,000,000
where
v1 is the value in period 1; p1 is the price per house in period 1; q1 is the quantity in period 1;
v2 is the value in period 2; p2 is the price per house in period 2; q2 is the quantity in period 2.
The ratio between the prices in the two periods, p2 and p1 ($250,000/$200,000 = 1.25) is the price relative (= p2/p1)
4.18 It is only necessary to have observations on two of the three components of equation 4.1 in order to analyse contributions to change in the value. If, for example, observations were only available on value and price, estimates of the quantity could be derived by dividing the value observations by price.
4.19 Now consider the case of price and quantity (and value) observations for houses in many suburbs. The quantities and prices of houses sold in different suburbs are likely to show different movements between periods. Answers are required to questions like: ‘what is the change over time in the quantity of houses sold; and what has been the contribution of price changes in the various suburbs to changes in the value of all houses over time?’ Answering these questions is the task of index numbers - to summarise the information on sets of prices and quantities into single measures to assist in understanding and analysing changes.
4.20 In essence, an index number is an average of either prices or quantities compared with the corresponding average in a base period. The problem is how to calculate the average.
4.21 More formally, the price index problem is how to derive numbers IP (an index of price) and IQ (an index of quantity) such that the product of the two is the change in the total value of the items between the base period (0) and any other period (t), that is
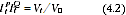
where Vt is the value of all items in period t and V0 is their value in period 0 (the base period). Based on equation 4.1, Vt can be represented as:
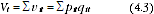
that is, the sum of the product of prices and quantities of each item denoted by subscript i. The summation range (i = 1.....n) is not shown in order to make the formula more readable.
Major index formulas
4.22 One widely used class of price indexes is obtained by defining the index as the percentage change between the periods compared in the total cost of producing (or purchasing) a fixed set of quantities, generally described as a “basket.” The meaning of such an index is easy to grasp and to explain to users.
4.23 In presenting index number formulas a simple starting point is to compare two sets of prices (sometimes called bilateral indexes). Consider price movements between two time periods, where the first period shall be denoted as period 0 and the second period as period t (period 0 occurs before period t). In order to calculate the price index, the quantities need to be held fixed at some point in time. The initial question is what period should be used to determine the basket (or quantities). The options are to use:
(1) The quantities of the first or earlier period;
(2) The quantities of the second (or more recent) period; or
(3) A combination (or average) of quantities in both periods
4.24 The first approach answers the question ‘how much would it cost in the second period, relative to the first period, to purchase the same bundle of goods and services as purchased in the first period?’ Estimating the cost of the basket in the second period’s prices simply requires multiplying the quantities of items purchased in the first period by the prices that prevailed in the second period. A price index is obtained from the ratio of the revalued basket to the total price of the basket in the first period. This approach was proposed by Laspeyres in 1871 and is referred to as a Laspeyres price index. It may be represented, with a base of 100.0, as

4.25 Approach two above is referred to as a Paasche price index and it answers the question 'how much would it have cost in the first period, relative to the second period, to purchase the same basket as was purchased in the second period'. The third approach above, a combination of the other two approaches, is used in the Fisher Ideal price index and the Törnqvist price index. These types of indexes are described in greater detail in the CPI and PPI Concepts, Sources and Methods publications.
4.26 When the periods being compared are far apart (e.g. over a long time series), Laspeyres and Paasche indexes can show a quite large divergence due to the fact that by using current quantities, the Paasche index can reflect substitution behaviour (e.g. in a consumer price index, consumers will shift consumption away from expensive items over time). The Fisher Ideal and Törnqvist indexes try to overcome some of the inherent difficulties of using a set of quantities fixed at either the earlier or the more recent period. In the absence of any firm indication that either period is better to use, then a combination of the two is a sensible compromise.
4.27 In practice, the Laspeyres formula has the advantage that the index can be extended to include another period’s price observations when available, as the weights are held fixed at some earlier base period. Therefore, only prices have to be collected on a regular basis. On the other hand, the Paasche, Fisher, and Törnqvist price indexes require collection of both current period price observations and current period weights before the index can be extended. It is much less costly and time consuming to calculate a time series Laspeyres index than a time series of Paasche, Fisher, and Törnqvist price indexes.
4.28 The Laspeyres formula is expressed above in terms of quantities and prices. In practice quantities might not be observable or meaningful for some price indexes (for example, how would the quantities of legal services, public transport and education be measured?). Thus in practice the Laspeyres formula can be estimated using value shares to weight price relatives - this is numerically equivalent to the formula 4.4 above.
4.29 To derive the price relatives form of the Laspeyres index, multiply the numerator of equation 4.4 by pi0/pi0 and rearrange as follows:

The term in parenthesis in equation 4.7 represents the value share of item i in the index reference (or commonly labelled, base) period, wi0. Let:

then the Laspeyres formula may be expressed as:

4.30 The important point to note here is that if price relatives are used then value weights (or value shares) must also be used. On the other hand, if prices are used directly rather than in their relative form, then the weights must be quantities.
Generating index series over more than two periods
4.31 Most users of price indexes require a continuous series of index numbers at specific time intervals. There are two options for applying the above formulae when compiling a price index series:
(i) Select one period as the base and separately calculate the movement between that period and each other period, which is called a fixed base or direct index; or
(ii) Calculate the period to period movements and chain link these (i.e. calculate the movement from the first period to the second, the second to the third with the movement from the first period to the third obtained as the product of these two movements).
4.32 The use of fixed weights (as in a Laspeyres type formula) over an extended period of time is not a sound index construction practice. For example, weights in the CPI have to be changed to reflect changes in the expenditure patterns of households over time.
4.33 There are two options in these situations if a fixed-weight index is used. One is to hold the weights constant over as long a period as seems reasonable, starting a new index each time the weights are changed. This means that a longer-term series is not available. The second is to update the weights more frequently and to chain link to produce a long-term series. The latter is the more common practice.
THE APPROACH USED IN THE HOUSE PRICE INDEX
4.34 In the discussion above it was shown how various price index formulae reflect different options as to the period in which the quantities are determined.
4.35 The approach used in HPI is that of the Laspeyres index. Being a Laspeyres index, the quantities underlying the HPI are fixed in the index reference period or weight reference period. An adaptation of the price relative form of the Laspeyres index is used in the HPI, however because quantities are observable, the first form of the formula 4.7 can be used, rather than the second form involving the value share, formula 4.9.
4.36 HPI employs a stratification approach to deal with the issue of changes in the composition of houses sold every quarter. In this approach houses are grouped into clusters. The item i in the index formulae corresponds to individual clusters (e.g. there are 11 clusters in Adelaide), rather than individual houses.
4.37 Because the purpose of the HPI is to measure changes in the price of the housing stock, the quantities used are the total housing stock in the weight reference period, rather than the number of sales in this period. The quantity for each item/cluster in the index is the total number of houses in the cluster.
4.38 The price relative for a cluster is calculated from the ratio of the median price of individual observations for that cluster in the current period to the median price in the previous period. As all houses are not transacted in each period, the prices attained from the sales of houses are used to represent the prices of all houses.
4.39 A Laspeyres index is being used for the HPI as it is not practical to calculate current weights every period (as required by other methods) - the current period housing stock is not readily available.
4.40 The weights for the HPI are updated periodically to reflect changes in the stock of houses, and indexes produced with the new weights are chain linked to those produced with the earlier weights.
4.41 Following chapters in this publication provide more information on how some of the concepts described above are applied in practice to the HPI. For example, clusters of houses are the item in the price index formula for which price change is measured every quarter: Chapter 5 describes how the cluster is a feature of a method adopted for dealing with changes in the composition of houses sold every quarter. Chapter 7 provides more information on weights used in the HPI and their sources. Chapters 8 and 9 provide information on the sources of price information, and on the median price, which is the particular form of the price observation used in HPI calculation. Chapter 10 provides more information on chain linking and Chapter 11 brings all this together to describe how the HPI is calculated in practice.
 Print Page
Print Page
 Print All
Print All