|
|
Technical Note: Data Quality
ESTIMATION PROCEDURE
1 Estimates derived from this survey were obtained by adjusting the MPS selection weights to account for the survey taking a subsample of the dwellings selected for the MPS. The weights were then adjusted to ensure that the survey estimates conformed to an independently estimated distribution of the population (by number of adults and children within the household, and by part of the state) rather than the distribution among respondents.
2 The estimates were then obtained by summing the weights of persons with the characteristic of interest. For example, an estimate of the total number of persons living in a dwelling with four bedrooms is obtained by adding together the weight for each person in the sample living in a dwelling with four bedrooms.
RELIABILITY OF ESTIMATES
3 Estimates in this publication are subject to non-sampling and sampling errors.
Non-sampling errors
4 Non-sampling errors may arise as a result of errors in the reporting, recording or processing of the data and can occur even if there is a complete enumeration of the population. Non-sampling errors can be introduced through inadequacies in the questionnaire, non-response, inaccurate reporting by respondents, errors in the application of survey procedures, incorrect recording of answers, and errors in data entry and processing.
5 It is difficult to measure the size of the non-sampling errors and the extent of these errors could vary considerably from survey to survey and from question to question. Every effort was made in the design of this survey and in the development of survey procedures to minimise the effect of these errors.
Sampling errors
6 Sampling error is the difference between the published estimate, calculated from a sample of dwellings, and the value that would have been produced if all dwellings had been included in the survey.
ESTIMATES OF SAMPLING ERRORS
7 One measure of the likely difference between a survey estimate and the 'true' population value is given by the Standard Error (SE). There are about two chances in three (67%) that a survey estimate is within one SE of the figure that would have been obtained if all households had been included in the survey, and about nineteen chances in twenty (95%) that the estimate lies within two SEs.
8 Due to space limitations, it is impractical to print the SE of each estimate in the publication. Instead, a table of SEs is provided to enable readers to determine the SE for an estimate based on the size of that estimate (see table T1). The SE table is derived from a mathematical model, which is created using the data collected in the survey. The figures in the SE table will not give a precise measure of the SE for a particular estimate but will provide an indication of its magnitude.
9 Linear interpolation can be used to calculate the SE of estimates falling between the sizes of estimates presented in table T1, using the following general formula:
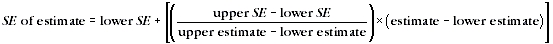
10 An example of the calculation and use of SEs is as follows. Table 2 shows that the estimated number of persons in WA that lived in a dwelling with four bedrooms was 611,200. Since this estimate is between 500,000 and 1,000,000, table T1 shows that the SE will lie between 13,231 and 16,980. The approximate value of the SE can be interpolated as follows:
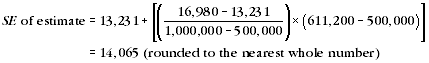
11 Therefore, there are about two chances in three that the true number of persons in WA that lived in a dwelling with four bedrooms lies between 597,135 and 625,265, and there are about nineteen chances in twenty that the value lies between 583,070 and 639,330.
12 The SE can also be expressed as a percentage of the estimate, known as the Relative Standard Error (RSE). The RSE is calculated by dividing the SE of an estimate by the estimate, and expressing it as a percentage. That is:
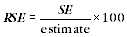
13 For example, the RSE for the number of persons that lived in a dwelling with four bedrooms is:
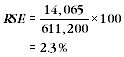
14 In general, the size of the SE increases as the size of the estimate increases. Conversely, the RSE decreases as the size of the estimate increases. Very small estimates are thus subject to high RSEs and are considered unreliable for general use.
15 Proportions and percentages formed from the ratio of two estimates are also subject to sampling errors. The size of the error depends on the accuracy of both the numerator and the denominator. A formula to approximate the RSE of a proportion is given below. This formula is only valid when the numerator is a subset of the denominator.
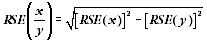
16 Published estimates are sometimes used to calculate the difference between two survey estimates. Such estimates are also subject to sampling error. The sampling error of the difference between two estimates depends on the SE of each estimate and the relationship (correlation) between them. The approximate SE of the difference between two estimates may be calculated using the following formula:
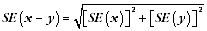
17 While this formula will only be exact for differences between separate and uncorrelated characteristics or subpopulations, it is expected to provide a good approximation for all differences likely to be of interest in this publication.
18 For example, Table 2 shows that an estimated 143,300 persons in WA lived in a dwelling with two bedrooms and 611,200 persons lived in a dwelling with four bedrooms. This equates to a difference of 467,900 persons. The standard error for each estimate is calculated using linear interpolation (as described above) and then the standard error on the estimate of the difference is calculated as:
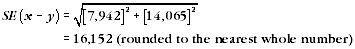
19 Therefore, there are about two chances in three that the true difference between the number of persons in WA living a dwelling with two or four bedrooms lies between 451,748 and 484,052, and there are about nineteen chances in twenty that the value lies between 435,596 and 500,204.
T1 STANDARD ERRORS ON ESTIMATES OF WA PERSONS |
|  |
 | SE | RSE |  |
Size of estimate
no. | no. | % |  |
|  |
| 1,000 | 649 | 64.9 |  |
| 1,500 | 824 | 55.0 |  |
| 2,000 | 973 | 48.7 |  |
| 2,500 | 1,105 | 44.2 |  |
| 3,000 | 1,224 | 40.8 |  |
| 3,500 | 1,334 | 38.1 |  |
| 4,000 | 1,435 | 35.9 |  |
| 5,000 | 1,620 | 32.4 |  |
| 8,000 | 2,080 | 26.0 |  |
| 10,000 | 2,335 | 23.3 |  |
| 20,000 | 3,306 | 16.5 |  |
| 30,000 | 4,020 | 13.4 |  |
| 50,000 | 5,098 | 10.2 |  |
| 100,000 | 6,932 | 6.9 |  |
| 200,000 | 9,264 | 4.6 |  |
| 300,000 | 10,887 | 3.6 |  |
| 500,000 | 13,231 | 2.6 |  |
| 1,000,000 | 16,980 | 1.7 |  |
| 2,000,000 | 21,414 | 1.1 |  |
|  |
20 Where differences between data items have been noted in the Summary of Findings, they are statistically significant unless otherwise specified. In this publication a statistically significant difference is one where there are nineteen chances in twenty that the difference noted reflects a true difference between population groups of interest rather than being the result of sampling variability.
|
|
 Print Page
Print Page
 Print All
Print All